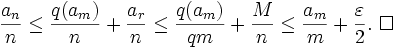SolSept0911-2

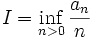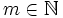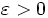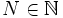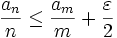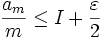Let $I = \inf_{n > 0} \frac{a_n}{n}$ . We show that for any $m \in \mathbb{N}$ , any $\varepsilon > 0$ , there is an $N \in \mathbb{N}$ with $\frac{a_n}{n} \le \frac{a_m}{m} + \frac{\varepsilon}{2}$ for all $n > N$ . By choosing $m$ so that $\frac{a_m}{m} \le I + \frac{\varepsilon}{2}$ , we get that 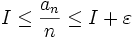$I \le \frac{a_n}{n} \le I + \varepsilon$ , and hence $\lim_{n \to \infty} \frac{a_n}{n} = I$ as claimed.

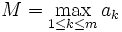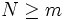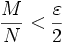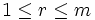Let $M = \max_{1 \le k \le m} a_k$ , and choose $N \ge m$ so that $\frac{M}{N} < \frac{\varepsilon}{2}$ . Let $n > N$ . By long division, we can write $n = q m + r$ , where $1 \le r \le m$ , and since $n > m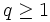$ , $q \ge 1$ . Then 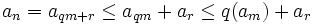$a_n = a_{qm + r} \le a_{qm} + a_r \le q(a_m) + a_r$ (by repeated applications of subadditivity), so

$\frac{a_n}{n} \le \frac{q(a_m)}{n} + \frac{a_r}{n} \le \frac{q(a_m)}{qm} + \frac{M}{n} \le \frac{a_m}{m} + \frac{\varepsilon}{2}.\ \square$

Retrieved from "http://www.efnet-math.org/w/SolSept0911-2"