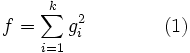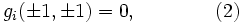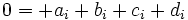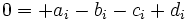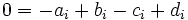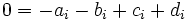Solution March 30, 2007
Problem
Let 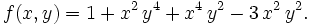
Determine whether there exist polynomials gi(x,y) with real coefficients such that
or argue that such a representation is not possible.
Solution
We will have to resort to arguing. Assume without loss of generality that 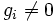 . Let
. Let 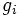 be fully expanded to sums of terms of the form
be fully expanded to sums of terms of the form 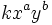 with
with 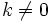 .
.
Note that 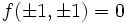 , so
, so
because a sum of squares of real numbers is zero if and only if all the numbers are zero.
If 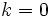 we'd have
we'd have 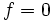 . So
. So 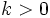 .
.
Let 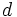 be the maximum degree of the
be the maximum degree of the 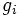 . Pick the smallest
. Pick the smallest 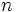 such that there is a term
such that there is a term 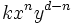 in one of the
in one of the 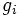 . Then, by comparing coefficients in (1), we find that
. Then, by comparing coefficients in (1), we find that 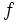 contains a term of the form
contains a term of the form 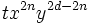 with
with 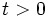 (
(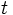 is the sum of at least one non-zero square, and thus positive), so
is the sum of at least one non-zero square, and thus positive), so 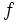 has degree at least
has degree at least 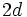 .
.
Therefore, the degree of 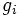 is at most 3.
is at most 3.
By a similar argument, 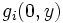 and
and 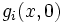 must be constant, because
must be constant, because 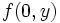 and
and 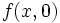 are constant polynomials.
are constant polynomials.
This means that 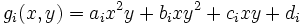 (what we just said about
(what we just said about 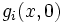 and
and 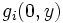 means that the coefficients for
means that the coefficients for 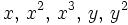 and
and 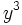 are zero) for some real numbers
are zero) for some real numbers 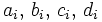 . (2) is then equivalent to the following system of linear equations:
. (2) is then equivalent to the following system of linear equations:
Solving these we find 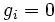 , contradicting our assumptions.
, contradicting our assumptions.