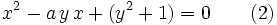Solution Nov. 30, 2006

Solution by int-e. Editing by landen.

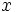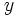Let $x\,$ and $y\,$ be positive integers such that 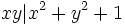$xy|x^2 +y^2 +1\,$ . Show that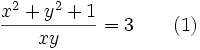 $\frac{x^2+y^2+1}{xy}=3\qquad (1)$

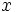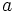This is equivalent to a quadratic equation in $x\,$ with $a\,$ being the quotient. We want to show that $a=3\,$ :

$x^2 -a\,y\,x + (y^2 + 1) = 0\qquad (2)$

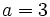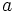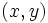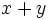Holding $a\,$ fixed there is a pair $(x,y)\,$ with $x+y\,$ as small as possible. By symmetry we can take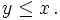 $y\le x\, .$

The quadratic in 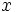$x\,$ has the product of its roots 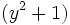$(y^2 + 1)\,$ . This means that 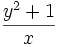$\frac{y^2+1}{x}$ is also in a solution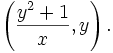 $\left( \frac{y^2+1}{x},y\right).$

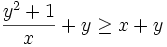$\frac{y^2+1}{x}+ y\ge x+y\,$ by the minimality of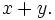 $x+y.\,$

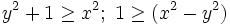$y^2+1\ge x^2;\ 1\ge(x^2-y^2)\,$ has the only solution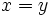 $x=y\,$

Then putting 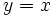$y=x\,$ in (2) gives:

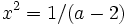$x^2 = 1/(a-2)\,$ which has the solution 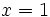$x=1\,$ and $a=3\,.\qquad\qquad \square$

Retrieved from "http://www.efnet-math.org/w/Solution_Nov._30%2C_2006"