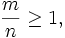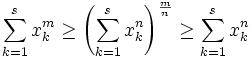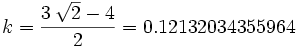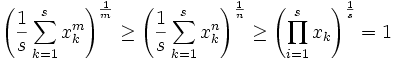Solution for Oct. 13, 2006
I.
Find the biggest real number  that for each right-angled triangle with sides
that for each right-angled triangle with sides 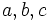 :
:
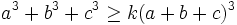 ;
; 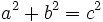
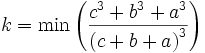
Let 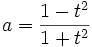 ;
;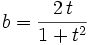 ;
;  This is justified because the expression to be minimized and the constraint are homogeneous.
This is justified because the expression to be minimized and the constraint are homogeneous.
We want to minimize 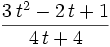 . Taking the derivative and setting to
. Taking the derivative and setting to 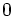 we get:
we get:
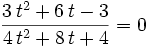 This has a single positive root at:
This has a single positive root at: 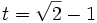

II.
For positive numbers 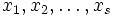 , we know that
, we know that 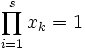 . Prove that for each
. Prove that for each 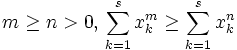
Using the generalized power mean, (http://en.wikipedia.org/wiki/Generalized_mean) we have:
Raising to the 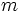 th power and multiplying by s.
th power and multiplying by s.
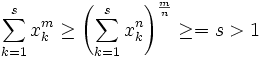
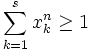 follows and since
follows and since